Difference between revisions of "Solving sparse systems"
From Medusa: Coordinate Free Mehless Method implementation
| Line 8: | Line 8: | ||
* direct: https://www.mathworks.com/help/matlab/ref/mldivide.html#bt42omx_head | * direct: https://www.mathworks.com/help/matlab/ref/mldivide.html#bt42omx_head | ||
* iterative: https://www.mathworks.com/help/matlab/math/systems-of-linear-equations.html#brzoiix, including bicgstab, gmres | * iterative: https://www.mathworks.com/help/matlab/math/systems-of-linear-equations.html#brzoiix, including bicgstab, gmres | ||
| + | |||
| + | |||
| + | <figure id="fig:meshless1"> | ||
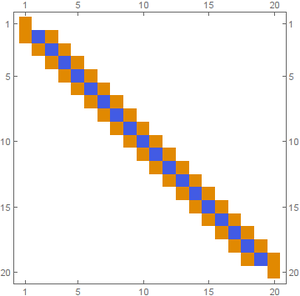
| + | [[File:matrix.png|300px|thumb|upright=2|alt=Matrix of the discretized PDE.|<caption>Matrix of the discretized PDE. </caption>]] | ||
| + | </figure> | ||
Eigen has the following methods: (https://eigen.tuxfamily.org/dox-devel/group__TopicSparseSystems.html) | Eigen has the following methods: (https://eigen.tuxfamily.org/dox-devel/group__TopicSparseSystems.html) | ||
| Line 14: | Line 19: | ||
Solving a simple sparse system $A x = b$ for steady space of heat equation in 1d with $n$ nodes, results in a matrix shown in fig. 1. | Solving a simple sparse system $A x = b$ for steady space of heat equation in 1d with $n$ nodes, results in a matrix shown in fig. 1. | ||
| − | |||
| − | |||
| − | |||
| − | |||
The following timings of solvers are given in seconds: | The following timings of solvers are given in seconds: | ||
Revision as of 14:07, 16 March 2017
There are many methods available for solving sparse systems. We compare some of them here.
Mathematica has the following methods available (https://reference.wolfram.com/language/ref/LinearSolve.html#DetailsAndOptions)
- direct: banded, cholesky, multifrontal (direct sparse LU)
- iterative: Krylov
Matlab has the following methods:
- direct: https://www.mathworks.com/help/matlab/ref/mldivide.html#bt42omx_head
- iterative: https://www.mathworks.com/help/matlab/math/systems-of-linear-equations.html#brzoiix, including bicgstab, gmres
Eigen has the following methods: (https://eigen.tuxfamily.org/dox-devel/group__TopicSparseSystems.html)
- direct: sparse LU
- iterative: bicgstab, cg
Solving a simple sparse system $A x = b$ for steady space of heat equation in 1d with $n$ nodes, results in a matrix shown in fig. 1.
The following timings of solvers are given in seconds:
| $n = 10^6$ | Matlab | Mathematica | Eigen |
|---|---|---|---|
| Banded | 0.16 | 0.28 | 0.04 |
| SparseLU | / | 1.73 | 0.82 |
| BICGStab / Krylov | 0.33 | 0.39 | 0.53 |
Incomplete LU preconditioner was used for BICGStab. Without the preconditioner BICGStab does not converge.